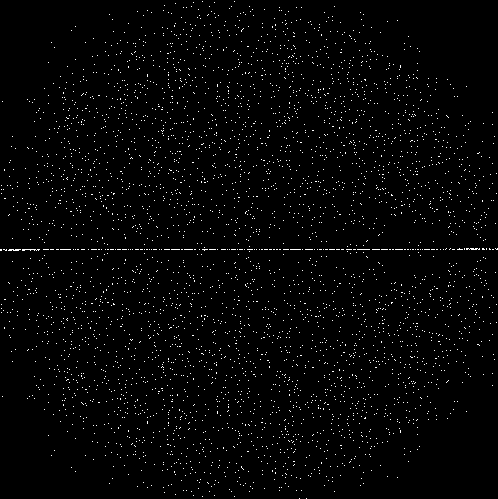
Fill an n×n matrix with independent and identically distributed values with variance n and mean 0.
Now plot the eigenvalues in the complex plane. As n → ∞, the eigenvalues become uniformly distributed in the complex unit disc!
For small n, there is a concentration along the real line, but this disappears as n → ∞. For this visualisation I've used 100 iterations of n = 50.
